|
Collisional Interaction of the Ring Current and the Plasmasphere
In the region of overlap between the ring
current and the plasmasphere, two plasma populations interact
with each other via multiple processes, such as wave-particle
interactions and Coulomb collisions. Energy of the ring current
ions is a source of free energy to excite plasma waves. This
energy, in turn, will be redistributed amongst the thermal and
energetic populations as the plasma waves undergo damping. Energy
contained in the ring current can also be transferred to the
plasmasphere through Coulomb collisions. In the following, our
previous results on the coupling between the ring current ions
and the plasmasphere through Coulomb collisions are presented.
In solving the kinetic equation (1), the
method of fractional step is used to decompose this drift-loss
model and only one process is solved at each fractional time
step. The instantaneous heating rate from the ring current to the
plasmaspheric species p is given by
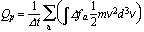 (5) (5)
where Dfa is the change of fa in Dt due to
Coulomb collisions with species p. We calculate the volume
heating rate to the thermal ions in the plasmasphere during the
recovery phase of a modeled major storm similar to that which
occurred in early February 1986 [Fok et al., 1995b]. Plate
2 shows the volume heating rate to the thermal ions at the
equator (left panels) and the noon-midnight meridian (right
panels) at (a) 2 and (b) 52 hours after the start of the recovery
phase, together with the corresponding Kp values. At 2
hours, the maximum heating rate is on the order of 1 eVcm-3s-1 and is located
at L shell of 2 to 2.5, with local time extending from
midnight to dawn. The region of high heating rate corresponds to
the location of peak density of low-energy (< 10 keV) ring
current ions, which provide the main contribution to the
plasmaspheric heating. This region of high heating rate drifts
eastward and reaches noon in the next few hours. The isocontours
of heating rate at L shells larger than 3 roughly follow
the thermal density calculated from the model of Rasmussen et
al. [1993]. The plasmasphere bulge located between noon and
dusk during this active period can be inferred in the equatorial
view of Plate 2a. The meridian view shows that the heating rate
peaks at the equator near the inner edge of the ring current and
is fairly uniform along field lines at high L shells. The
localized heating near the Earth is a consequence of ring current
ions which have an anisotropic pitch-angle distribution (peaks at
90°) caused by strong charge exchange loss at low L
shells.
In late recovery (Plate 2b), isocontours of
heating rate expand and fall off with L smoothly as a
result of the refilling of the plasmasphere during the storm
recovery. The high heating rate (on the order of 1 eVcm-3s-1), which is seen
at L < 2.5 at 2 hours, is diminished due to the charge
exchange losses of low-energy (< 10 keV) ions at that
location. In contrast, the heating rates at high L's are
higher at late recovery than at early recovery. In the meridian
view, the peak heating rate at the equator is more pronounced and
extends to higher L shells as a consequence of strong ring
current pitch-angle anisotropy during late recovery of the storm
[Fok et al., 1995a].
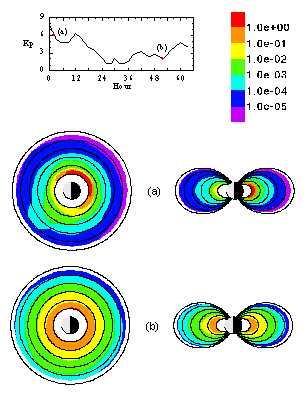
Plate 2. Plasmaspheric ion volume
heating rate (eVcm-3s-1) from the ring current at the equator (left) and
the noon-midnight meridian (right) at (a) 2 hours and (b) 52
hours after the main phase of the model storm.
Plasmaspheric Response to the Heating
from the Ring Current
In order to see the plasmaspheric response
to the heating from the energetic ions, the thermal ion
temperatures are calculated using the heat source generated from
our model for the February 1986 storm as input to the FLIP model.
The volume heating rate calculated from our model is scaled by
the ratio of plasmaspheric densities obtained from the model of Rasmussen
et al. [1993] and FLIP. We modified the standard FLIP model
slightly in order to accommodate direct ion heating from the ring
current source.
The altitude profiles of ion temperature at
L = 4, on the morning and evening side, at different
elapsed times during the storm recovery as calculated from FLIP
are plotted in Figure 3. In both cases, heating from the ring
current increases the ion temperature at altitudes above the heat
sink due to the neutral atmosphere, about 500 km. The ion
temperature is increasing throughout the recovery until t
~ 50 hours after which it decreases. There is a two- to five-fold
increase in temperature compared with no heating from ring
current. For comparison, the mean DE 1/RIMS H+ temperatures
during October and November of 1981 are also shown. In general,
heating from the ring current can more than account for the ion
temperature observed. we have clearly shown that the enhanced ion
temperature in the plasmasphere cannot be explained without the
heating from a magnetospheric source.
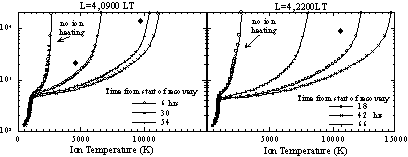
Figure 3. Altitude profiles of ion
temperatures at L = 4, morning (left) and evening(right)
at different times relative to the start of the recovery. Mean DE
1/RIMS data in 1981 are shown with the ®.
Back to Science Main
|
